1 The Maxwellian distribution function

The thermal distribution function is expressed in its general relativistic form

(2)

where Θ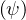 = T_e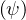∕m_ec² is the ratio of the local electron temperature T_e 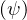to the electron rest mass energy m_ec² while the relativistic Lorentz correction factor is defined as γ² = p² + 1. Here, ξ is the cosine of the pitch angle. By symmetry, the Maxwellian distribution function is independant of ξ. By definition, f_M is normalized to unity in the interval p ∈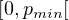, where p_min is a given upper cut-off limit for the Maxwellian. The parameter α_M is then given by the integral

(3)

Recalling that γdγ = pdp,

(4)

where

(5)

By integrating by parts

For Θ ≪ 1, using the large argument asymptotic development

(9)

the usual expression keeping the first in the expansion

(12)

In the LUKE code, the momentum p here expressed in relativistic units m_ec is normalized to the thermal reference value p_th^† = m_ev_th^†,

(13)

and consequently

(14)

since thermal electrons are only weakly relativistic. The well known relativistic Lorentz correction factor γ is then simply given by the relation

(15)

and in the non-relativistic limit, i.e. the condition p²β_th^†2 ≪ 1 or γ ≈ 1 holds.

Since in relativistic units, the total energy is linked to the relativistic momentum by the expression,

(16)

it is straightforward to express the kinetic energy E_c as a function of p in units of electron rest mass energy m_ec²

(17)

Finally, concerning the normalization of the electron velocity v, one has

(21)

or

(22)

with

(23)

Consequently, the non-relativistic expression of f_M is only valid when γ ≃ 1, or in an equivalent form

(24)

Since p may be as large as 30 in numerical calculations, in order to correctly describe momentum dynamics of the fastest electrons, it results that

(25)

or

(26)

since β_th^† = and Θ^† = T_e^†∕m_ec². Therefore, in a thermonuclear plasma, one must always consider the relativistic form of the Maxwellian distribution function. In addition, considering the asymptotic limit of K₂ for large arguments (??), the condition is

(27)

or

which is always satisfied, since T_e^† never exceeds a few ten keV in tokamak plasmas. This means that the approximate formulation of f is fully valid.

In normalized units,

(30)

since p_th^† = β_th^†, with

(31)

and the modified normalisation coefficient becomes

(32)

The Maxwellian distribution function be expressed in an alternative form, useful for calculating interpolation between full and half-grids,

(33)

One can then easily cross-check that

(34)

is well recovered.